Subspace-based steady-state dynamic analysis | ||
| ||
ProductsAbaqus/StandardAbaqus/CAE
Introduction
Steady-state dynamic analysis provides the steady-state amplitude and phase of the response of a system subjected to harmonic excitation at a given frequency. Usually such analysis is done as a frequency sweep, by applying the loading at a series of different frequencies and recording the response. In Abaqus/Standard the subspace-based steady-state dynamic analysis procedure is used to conduct the frequency sweep.
In a subspace-based steady-state dynamic analysis the response is based on direct solution of the steady-state dynamic equations projected onto a subspace of modes. The modes of the undamped, symmetric system must first be extracted using the eigenfrequency extraction procedure. The modes will include eigenmodes and, if activated in the eigenfrequency extraction step, residual modes. The procedure is based on the assumption that the forced steady-state vibration can be represented accurately by a number of modes of the undamped system that are in the range of the excitation frequencies of interest. The number of modes extracted must be sufficient to model the dynamic response of the system adequately, which is a matter of judgment on your part. The projection of the dynamic equilibrium equations onto a subspace of selected modes leads to a small system of complex equations that is solved for modal amplitudes, which are then used to compute nodal displacements, stresses, etc.
When defining a subspace-based steady-state dynamic step, you specify the frequency ranges of interest and the number of frequencies at which results are required in each range (including the bounding frequencies of the range). In addition, you can specify the type of frequency spacing (linear or logarithmic) to be used, as described below (Selecting the frequency spacing). Logarithmic frequency spacing is the default if the frequency ranges are specified directly or by eigenfrequencies. If the frequency ranges are specified by the frequency spread, only linear spacing can be used. Frequencies should be given in cycles/time.
The frequency points for which results are required can be spaced equally along the frequency axis (on a linear or a logarithmic scale), or they can be biased toward the ends of the user-defined frequency range by introducing a bias parameter (see The bias parameter below).
The subspace-based steady-state dynamic analysis procedure can be used:
for nonsymmetric stiffness;
when any form of damping (except modal damping) is included; and
when viscoelastic material properties must be taken into account.
While the response in this procedure is for linear vibrations, the prior response can be nonlinear. Initial stress effects (stress stiffening) will be included in the steady-state dynamic response if nonlinear geometric effects (General and perturbation procedures) were included in any general analysis step prior to the eigenfrequency extraction step preceding the subspace-based steady-state dynamic procedure.
Input File Usage
STEADY STATE DYNAMICS, SUBSPACE PROJECTION
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace
Ignoring damping
If damping terms can be ignored, you can specify that a real, rather than a complex, system matrix be generated and projected, which can significantly reduce computational time, at the cost of ignoring the damping effects.
Input File Usage
STEADY STATE DYNAMICS, SUBSPACE PROJECTION, REAL ONLY
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace: Compute real response only
Selecting the type of frequency interval for which output is requested
Three types of frequency intervals are permitted for output from a subspace-based steady-state dynamic step.
Specifying the frequency ranges by using the system's eigenfrequencies
By default, the eigenfrequency type of frequency interval is used; in this case the following intervals exist in each frequency range:
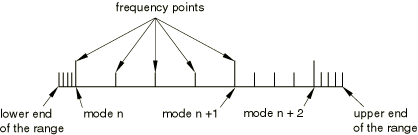
First interval: extends from the lower limit of the frequency range given to the first eigenfrequency in the range.
Intermediate intervals: extend from eigenfrequency to eigenfrequency.
Last interval: extends from the highest eigenfrequency in the range to the upper limit of the frequency range.
For each of these intervals the frequencies at which results are calculated are determined using the user-defined number of points (which includes the bounding frequencies for the interval) and the optional bias function (which is discussed below and allows the sampling points on the frequency scale to be spaced closer together at eigenfrequencies in the frequency range). Thus, detailed definition of the response close to resonance frequencies is allowed. Figure 1 illustrates the division of the frequency range for 5 calculation points and a bias parameter equal to 1.
Input File Usage
STEADY STATE DYNAMICS, SUBSPACE PROJECTION, INTERVAL=EIGENFREQUENCY
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace: Use eigenfrequencies to subdivide each frequency range
Specifying the frequency ranges by the frequency spread
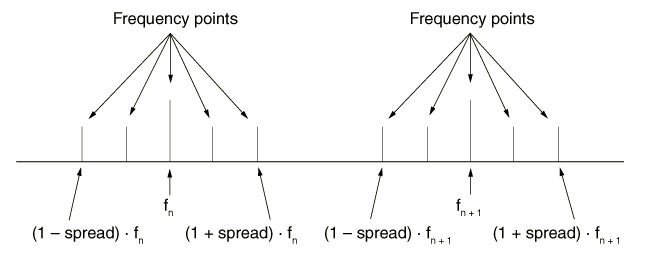
If the spread type of frequency interval is selected, intervals exist around each eigenfrequency in the frequency range. For each of the intervals the equally spaced frequencies at which results are calculated are determined using the user-defined number of points (which includes the bounding frequencies for the interval). The minimum number of frequency points is 3. If the user-defined value is less than 3 (or omitted), the default value of 3 points is assumed. Figure 2 illustrates the division of the frequency range for 5 calculation points.
The bias parameter is not supported with the spread type of frequency interval.
Input File Usage
STEADY STATE DYNAMICS, SUBSPACE PROJECTION, INTERVAL=SPREAD lwr_freq, upr_freq, numpts, bias_param, freq_scale_factor, spread
Abaqus/CAE Usage
You cannot specify frequency ranges by frequency spread in Abaqus/CAE.
Specifying the frequency ranges directly
If the alternative range type of frequency interval is chosen, there is only one interval in the specified frequency range spanning from the lower to the upper limit of the range. This interval is divided using the user-defined number of points and the optional bias function, which can be used to space the sampling frequency points closer to the range limits. For the range type of frequency interval, the peak responses around the system's eigenfrequencies may be missed since the sampling frequencies at which output will be reported will not be biased toward the eigenfrequencies.
Input File Usage
STEADY STATE DYNAMICS, SUBSPACE PROJECTION, INTERVAL=RANGE
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace: toggle off Use eigenfrequencies to subdivide each frequency range
Selecting the frequency spacing
Two types of frequency spacing are permitted for a subspace-based steady-state dynamic step. For the logarithmic frequency spacing (the default), the specified frequency ranges of interest are divided using a logarithmic scale. Alternatively, a linear frequency spacing can be used if a linear scale is desired.
Input File Usage
Use the following option to specify logarithmic frequency spacing:
STEADY STATE DYNAMICS, SUBSPACE PROJECTION, FREQUENCY SCALE=LOGARITHMIC (default)
Use the following option to specify linear frequency spacing:
STEADY STATE DYNAMICS, SUBSPACE PROJECTION, FREQUENCY SCALE=LINEAR
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace: Scale: Logarithmic or Linear
Requesting multiple frequency ranges
You can request multiple frequency ranges for a subspace-based steady-state dynamic step. When both frequency ranges and additional single frequency points are requested, the frequency ranges must be specified first.
Input File Usage
Repeat the data lines as often as necessary to request multiple frequency ranges or multiple single frequency points:
STEADY STATE DYNAMICS, SUBSPACE PROJECTION lwr_freq1, upr_freq1, numpts1, bias_param1, freq_scale_factor1 lwr_freq2, upr_freq2, numpts2, bias_param2, freq_scale_factor2 ... single_freq1 single_freq2 ...
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace: Data: enter data in table, and add rows as necessary
![]()
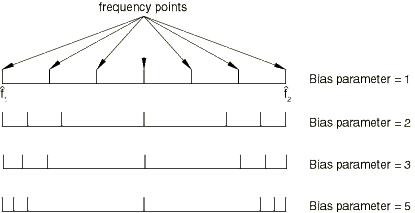
The bias parameter
The bias parameter can be used to provide closer spacing of the results points either toward the middle or toward the ends of each frequency interval. Figure 3 shows a few examples of the effect of the bias parameter on the frequency spacing.
The bias formula used in subspace-based steady-state dynamics is
where
- y
;
- n
is the number of frequency points at which results are to be given within a frequency interval (discussed above);
- k
is one such frequency point ();
is the lower limit of the frequency interval;
is the upper limit of the frequency interval;
is the frequency at which the kth results are given;
- p
is the bias parameter value; and
is the frequency or the logarithm of the frequency, depending on the value chosen for the frequency scale.
A bias parameter, p, that is greater than 1.0 provides closer spacing of the results points toward the ends of the frequency interval, while values of p that are less than 1.0 provide closer spacing toward the middle of the frequency interval. The default bias parameter is 3.0 for an eigenfrequency interval and 1.0 for a range frequency interval.
![]()
The frequency scale factor
The frequency scale factor can be used to scale frequency points. All the frequency points, except the lower and upper limit of the frequency range, are multiplied by this factor. This scale factor can be used only when the frequency interval is specified by using the system's eigenfrequencies (see Specifying the frequency ranges by using the system's eigenfrequencies above).
![]()
Damping
If damping is absent, the response of a structure will be unbounded if the forcing frequency is equal to an eigenfrequency of the structure. To get quantitatively accurate results, especially near natural frequencies, accurate specification of damping properties is essential. The various damping options available are discussed in Material damping.
In subspace-based steady-state dynamic analysis damping can be created by the following:
dashpots (see Dashpots),
“Rayleigh” damping associated with materials and elements (see Material damping),
structural damping (see Damping in dynamic analysis),
viscoelasticity included in the material definitions (see Frequency domain viscoelasticity),
contributions from infinite elements (see Infinite elements) or defined impedance conditions (see Acoustic and shock loads) on acoustic elements, and
“volumetric drag” (viscous Rayleigh damping) in acoustic elements (see Acoustic medium).
If you specify that a real-only system matrix be generated and projected (see Ignoring damping above), all forms of damping are ignored, including quiet boundaries on infinite elements and nonreflecting boundaries on acoustic elements.
![]()
Contact conditions with sliding friction
Abaqus/Standard automatically detects the contact nodes that are slipping due to velocity differences imposed by the motion of the reference frame or the transport velocity in prior steps. At those nodes the tangential degrees of freedom are not constrained and the effect of friction results in an unsymmetric contribution to the stiffness matrix. At other contact nodes the tangential degrees of freedom are constrained.
Friction at contact nodes at which a velocity differential is imposed can give rise to damping terms. There are two kinds of friction-induced damping effects. The first effect is caused by the friction forces stabilizing the vibrations in the direction perpendicular to the slip direction. This effect exists only in three-dimensional analysis. The second effect is caused by a velocity-dependent friction coefficient. If the friction coefficient decreases with velocity (which is usually the case), the effect is destabilizing and is also known as “negative damping.” For more details, see Coulomb friction. Subspace-based steady-state dynamics analysis allows you to include these friction-induced contributions to the damping matrix.
Input File Usage
STEADY STATE DYNAMICS, SUBSPACE PROJECTION, FRICTION DAMPING=YES
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace: Include friction-induced damping effects
![]()
Selecting the modes on which to project
You can select modes by specifying the mode numbers individually, by requesting that Abaqus/Standard generate the mode numbers automatically, or by requesting the modes that belong to specified frequency ranges. If you do not select the modes, all modes extracted in the prior eigenfrequency extraction step, including residual modes if they were activated, are used in the modal superposition.
Input File Usage
Use the following option to select the modes by specifying mode numbers individually:
SELECT EIGENMODES, DEFINITION=MODE NUMBERS
Use the following option to request that Abaqus/Standard generate the mode numbers automatically:
SELECT EIGENMODES, GENERATE, DEFINITION=MODE NUMBERS
Use the following option to select the modes by specifying a frequency range:
SELECT EIGENMODES, DEFINITION=FREQUENCY RANGE
Abaqus/CAE Usage
You cannot select the modes in Abaqus/CAE; all modes extracted are used in the modal superposition.
![]()
Selecting the subspace projection frequency
You can control the frequency of the subspace projections. By default, the dynamic equations are projected onto the subspace at each frequency you request. However, considerable computational savings can be obtained if the projection onto the subspace is performed only at selected frequency points.
Projecting the subspace at each frequency requested
By default, the dynamic equations are projected onto the subspace at each frequency you requested. This is the most computationally expensive method. If coupled acoustic-structural modes are extracted in the preceding eigenfrequency extraction step, this is the only method allowed.
Input File Usage
Use either of the following options:
STEADY STATE DYNAMICS, SUBSPACE PROJECTION STEADY STATE DYNAMICS, SUBSPACE PROJECTION=ALL FREQUENCIES
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace: Projection: Evaluate at each frequency
Projecting the subspace using model properties at the center frequency of all ranges
You can perform only one projection using model properties evaluated at the center frequency of all ranges and individual frequency points specified. The center frequency is determined on a logarithmic or linear scale depending on the spacing requested.
This method is the least expensive. However, it should be chosen only when the material properties do not depend strongly on frequency.
Input File Usage
STEADY STATE DYNAMICS, SUBSPACE PROJECTION=CONSTANT
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace: Projection: Constant
Projecting the subspace at each extracted eigenfrequency
You can perform the projections at each extracted eigenfrequency in the requested frequency range and at eigenfrequencies immediately outside the range. The projected mass, stiffness, and damping matrices are then interpolated at each frequency point requested. The interpolation is performed on a linear or logarithmic scale depending on the spacing requested.
Input File Usage
STEADY STATE DYNAMICS, SUBSPACE PROJECTION=EIGENFREQUENCY
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace: Projection: Interpolate at eigenfrequencies
Projecting the subspace based on material property changes as a function of frequency
You can select how often subspace projections are performed based on material property changes as a function of frequency. You specify the relative change in material stiffness and damping properties allowed before a new projection is performed. In the beginning of the subspace-based steady-state dynamic step Abaqus/Standard computes a table of relative changes in material stiffness and damping properties, and projections are performed based on the strictest of the two criteria. The projections are then interpolated at each requested frequency point as described above. The default value for the allowable stiffness or damping change is 0.1.
Input File Usage
STEADY STATE DYNAMICS, SUBSPACE PROJECTION=PROPERTY CHANGE, DAMPING CHANGE=percentage, STIFFNESS CHANGE=percentage
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace: Projection: As a function of property changes, Max. damping change: percentage, Max. stiffness change: percentage
Projecting the subspace at the limits of each frequency range
You can select how often subspace projections are performed based on the limits of each frequency range. The projections onto the modal subspace of the dynamic equations are performed at the lower limit of each frequency range and at the upper limit of the last frequency range. The interpolation of the projected mass, stiffness, and damping matrices is performed on a linear scale. This method can be used only with the SIM architecture.
This method should be chosen when the frequency dependence of material properties is close to linear within a frequency range.
Input File Usage
STEADY STATE DYNAMICS, SUBSPACE PROJECTION=RANGE
Abaqus/CAE Usage
Step module: Create Step: Linear perturbation: Steady-state dynamics, Subspace: Projection: Interpolate at lower and upper frequency limits
![]()
Initial conditions
The base state is the current state of the model at the end of the last general analysis step prior to the steady-state dynamic step. If the first step of an analysis is a perturbation step, the base state is determined from the initial conditions (Initial conditions in Abaqus/Standard and Abaqus/Explicit). Initial condition definitions that directly define solution variables, such as velocity, cannot be used in a steady-state dynamic analysis.
![]()
Boundary conditions
In a subspace-based steady-state dynamic analysis both the real and imaginary parts of any degree of freedom are either restrained or unrestrained; it is physically impossible to have one part restrained and the other part unrestrained. Abaqus/Standard will restrain both the real and imaginary parts of a degree of freedom automatically even if only one part is restrained.
Base motion
It is not possible to prescribe nonzero displacements and rotations directly as boundary conditions (Boundary conditions in Abaqus/Standard and Abaqus/Explicit) in subspace-based steady-state dynamic analysis. Instead, prescribed motion can be specified as base motion; nonzero displacement or acceleration history definitions given as boundary conditions are ignored, and any changes in the support conditions from the eigenfrequency extraction step are flagged as errors. The method for prescribing base motion in modal superposition procedures is described in Transient modal dynamic analysis.
Base motions can be defined by a displacement, a velocity, or an acceleration history. For an acoustic pressure the displacement is used to describe an acoustic pressure history. If the prescribed excitation record is given in the form of a displacement or velocity history, Abaqus/Standard differentiates it to obtain the acceleration history. The default is to give an acceleration history for mechanical degrees of freedom and to give a displacement for an acoustic pressure.
When secondary bases are used, low frequency eigenmodes are extracted for each “big” mass applied in the model. Use care when choosing the lower limit range for the frequency in such cases. The “big” mass modes are important in the modal superposition. However, you should not request the response at zero or an arbitrarily low frequency level because this forces Abaqus/Standard to calculate the responses at frequencies between these “big” mass eigenfrequencies, which is not desirable.
Frequency-dependent base motion
An amplitude definition can be used to specify the amplitude of a base motion as a function of frequency (Amplitude Curves).
Input File Usage
Use both of the following options:
AMPLITUDE, NAME=name BASE MOTION, REAL or IMAGINARY, AMPLITUDE=name
Abaqus/CAE Usage
Load module; Create Boundary Condition; Step: step_name; Category: Mechanical; Types for Selected Step: Displacement base motion or Velocity base motion or Acceleration base motion; Basic tabbed page: Degree-of-freedom: U1, U2, U3, UR1, UR2, or UR3; Amplitude: name
![]()
Loads
The following loads can be prescribed in a subspace-based steady-state dynamic analysis, as described in Concentrated loads:
Concentrated nodal forces can be applied to the displacement degrees of freedom (1–6).
Distributed pressure forces or body forces can be applied; the distributed load types available with particular elements are described in Abaqus Elements Guide.
Incident wave loads can be applied; see Acoustic and shock loads. Incident wave loads can be used to model sound waves from distinct planar or spherical sources or from diffuse fields.
These loads are assumed to vary sinusoidally with time over a user-specified range of frequencies. Loads are given in terms of their real and imaginary components.
Input File Usage
Use either of the following input lines to define the real (in-phase) part of the load:
CLOAD or DLOAD CLOAD or DLOAD, REAL
Use the following input line to define the imaginary (out-of-phase) part of the load:
CLOAD or DLOAD, IMAGINARY
Abaqus/CAE Usage
You can only define the real (in phase) part of the load in Abaqus/CAE.
Load module: load editor: real (in-phase) part
Frequency-dependent loading
An amplitude definition can be used to specify the amplitude of a load as a function of frequency (Amplitude Curves).
Input File Usage
Use both of the following options:
AMPLITUDE, NAME=name CLOAD or DLOAD, REAL or IMAGINARY, AMPLITUDE=name
Abaqus/CAE Usage
Load or Interaction module: Create Amplitude: Name:name
Load module: load editor: Amplitude:name
Loading limitations
Coriolis distributed loading adds an imaginary antisymmetric contribution to the overall system of equations. This contribution is currently accounted for in solid and truss elements only and is activated by requesting the unsymmetric matrix storage and solution scheme for the step.
Fluid flux loading cannot be used in subspace-based steady-state dynamic analysis.
![]()
Predefined fields
Predefined temperature fields can be specified in subspace-based steady-state dynamic analysis (see Predefined Fields) and will produce harmonically varying thermal strains if thermal expansion is included in the material definition (Thermal expansion). Other predefined fields are ignored.
![]()
Material options
As in any dynamic analysis procedure, mass or density (Density) must be assigned to some regions of any separate parts of the model where dynamic response is required. If an analysis is desired in which the inertia effects are neglected, the density should be set to a very small number. Natural damping, as well as individual dashpots, can be included in this procedure.
Viscoelastic effects can be included in subspace-based steady-state dynamic analysis. The linearized viscoelastic response is considered to be a perturbation about a nonlinear preloaded state, which is computed on the basis of purely elastic behavior (long-term response) in the viscoelastic components. Therefore, the vibration amplitude must be sufficiently small so that the material response in the dynamic phase of the problem can be treated as a linear perturbation about the predeformed state. Viscoelastic frequency domain response is described in Frequency domain viscoelasticity.
The following material properties are not active during subspace-based steady-state dynamic analyses: plasticity and other inelastic effects, thermal properties (except for thermal expansion), mass diffusion properties, electrical properties (except for the electrical potential, , in piezoelectric analysis), and pore fluid flow properties—see General and perturbation procedures.
Numerical investigations show that in general the accuracy of the results in the subspace-based steady-state dynamic step is improved if in the previous eigenfrequency extraction step the material properties are evaluated at a frequency in the vicinity of the center of the range spanned by the frequencies specified for the steady-state dynamic step (see Natural frequency extraction). In this case the modes extracted in the previous eigenfrequency extraction step for the undamped system will reflect most accurately the modes of the damped system at frequencies located in the proximity of the frequency at which the material properties are evaluated. Thus, if the steady-state dynamic response is sought for a large span of frequencies and the specified material properties vary significantly over this span, the results will be more accurate if the range is divided into smaller ranges and several separate analyses are run over these smaller ranges with the material properties evaluated at appropriate frequencies.
![]()
Elements
Any of the following elements available in Abaqus/Standard can be used in a subspace-based steady-state dynamic analysis:
stress/displacement elements (other than generalized axisymmetric elements with twist);
acoustic elements;
piezoelectric elements; and
hydrostatic fluid elements.
![]()
Output
In subspace-based steady-state dynamic analysis the value of an output variable such as strain (E) or stress (S) is a complex number with real and imaginary components. In the case of data file output the first printed line gives the real components while the second lists the imaginary components. Results and data file output variables are also provided to obtain the magnitude and phase of many variables (see Abaqus/Standard output variable identifiers). In this case the first printed line in the data file gives the magnitude while the second gives the phase angle.
The following variables are provided specifically for subspace-based steady-state dynamic analysis:
Element integration point variables:
- PHS
Magnitude and phase angle of all stress components.
- PHE
Magnitude and phase angle of all strain components.
- PHEPG
Magnitude and phase angles of the electrical potential gradient vector.
- PHEFL
Magnitude and phase angles of the electrical flux vector.
- PHMFL
Magnitude and phase angle of the mass flow rate in fluid link elements.
- PHMFT
Magnitude and phase angle of the total mass flow in fluid link elements.
For connector elements, the following element output variables are available:
- PHCTF
Magnitude and phase angle of connector total forces.
- PHCEF
Magnitude and phase angle of connector elastic forces.
- PHCVF
Magnitude and phase angle of connector viscous forces.
- PHCRF
Magnitude and phase angle of connector reaction forces.
- PHCSF
Magnitude and phase angle of connector friction forces.
- PHCU
Magnitude and phase angle of connector relative displacements.
- PHCCU
Magnitude and phase angle of connector constitutive displacements.
- PHCV
Magnitude and phase angle of connector relative velocities.
- PHCA
Magnitude and phase angle of connector relative accelerations.
Nodal variables:
- PU
Magnitude and phase angle of all displacement/rotation components at a node.
- PPOR
Magnitude and phase angle of the fluid or acoustic pressure at a node.
- PHPOT
Magnitude and phase angle of the electrical potential at a node.
- PRF
Magnitude and phase angle of all reaction forces/moments at a node.
- PHCHG
Magnitude and phase angle of the reactive charge at a node.
Neither element energy densities (such as the elastic strain energy density, SENER) nor whole element energies (such as the total kinetic energy of an element, ELKE) are available for output in a SIM-based, subspace-based steady-state dynamic analysis.
The standard output variables U, V, A, and the variable PU listed above correspond to motions relative to the motion of the primary base in a subspace-based steady-state dynamic analysis. Total values, which include the motion of the primary base, are also available:
- TU
Components of total displacement/rotation at a node.
- TV
Components of total velocity at a node.
- TA
Components of total acceleration at a node.
- PTU
Magnitude and phase angle of all total displacement/rotation components at a node.
The specified base motion is available for subspace-based steady-state dynamic analysis and can be output to the data, results, and/or output database files (see Output to the Data and Results Files and Output to the Output Database).
- BM
Base motion.
Whole model variables such as ALLIE (total strain energy) are available for subspace-based steady-state dynamic analysis as output to the data, results, and/or output database files (see Output to the Data and Results Files and Output to the Output Database).
Acoustic contribution factors
Computation of the acoustic contribution factors helps you determine the major noise sources. The procedure for computing the acoustic contribution factors is based on the modal analysis formulation of acoustic-structural problems with uncoupled modes. For more information, see Acoustic contribution factors in mode-based and subspace-based steady-state dynamic analyses.
![]()
Input file template
HEADING … AMPLITUDE, NAME=loadamp Data lines to define an amplitude curve as a function of frequency (cycles/time) AMPLITUDE, NAME=base Data lines to define an amplitude curve to be used to prescribe base motion ** STEP, NLGEOM Include the NLGEOM parameter so that stress stiffening effects will be included in the steady-state dynamics step STATIC **Any general analysis procedure can be used to preload the structure … CLOAD and/or DLOAD Data lines to prescribe preloads TEMPERATURE and/or FIELD Data lines to define values of predefined fields for preloading the structure BOUNDARY Data lines to specify boundary conditions to preload the structure END STEP ** STEP FREQUENCY Data line to control eigenvalue extraction BOUNDARY Data lines to assign degrees of freedom to the primary base BOUNDARY, BASE NAME=base2 Data lines to assign degrees of freedom to a secondary base END STEP ** STEP STEADY STATE DYNAMICS, SUBSPACE PROJECTION Data lines to specify frequency ranges and bias parameters SELECT EIGENMODES Data lines to define the applicable mode ranges BASE MOTION, DOF=dof, AMPLITUDE=base BASE MOTION, DOF=dof, AMPLITUDE=base, BASE NAME=base2 CLOAD and/or DLOAD, AMPLITUDE=loadamp Data lines to specify sinusoidally varying, frequency-dependent loads … END STEP