Damage initiation for ductile metals

The ductile criterion is a phenomenological model for predicting the onset of damage due to nucleation, growth, and coalescence of voids. The model assumes that the equivalent plastic strain at the onset of damage, ${\overline{\epsilon }}_{\mathrm{D}}^{pl}$, is a function of stress triaxiality and strain rate:

where $\eta =-p/q$ is the stress triaxiality, p is the pressure stress, q is the Mises equivalent stress, and ${\dot{\overline{\epsilon }}}^{pl}$ is the equivalent plastic strain rate. The criterion for damage initiation is met when the following condition is satisfied:

where ${\omega }_{\mathrm{D}}$ is a state variable that increases monotonically with plastic deformation. At each increment during the analysis the incremental increase in ${\omega }_{\mathrm{D}}$ is computed as

In Abaqus/Standard the ductile criterion can be used in conjunction with the Mises, Johnson-Cook, Hill, and Drucker-Prager plasticity models and in Abaqus/Explicit in conjunction with the Mises, Johnson-Cook, Hill, and Drucker-Prager plasticity models, including equation of state.

DAMAGE INITIATION, CRITERION=DUCTILE, DEPENDENCIES=n

DAMAGE INITIATION, CRITERION=DUCTILE, LODE DEPENDENT

DAMAGE INITIATION, CRITERION=JOHNSON COOK

The shear criterion is a phenomenological model for predicting the onset of damage due to shear band localization. The model assumes that the equivalent plastic strain at the onset of damage, ${\overline{\epsilon }}_{\mathrm{S}}^{pl}$, is a function of the shear stress ratio and strain rate:

Here ${\theta }_s=\left(q+k_{s}p\right)/{\tau }_{\max }$ is the shear stress ratio, ${\tau }_{\max }$ is the maximum shear stress, and $k_s$ is a material parameter. A typical value of $k_s$ for aluminum is $k_s$ = 0.3 (Hooputra et al., 2004). The criterion for damage initiation is met when the following condition is satisfied:

where ${\omega }_{\mathrm{S}}$ is a state variable that increases monotonically with plastic deformation proportional to the incremental change in equivalent plastic strain. At each increment during the analysis the incremental increase in ${\omega }_{\mathrm{S}}$ is computed as

In Abaqus/Explicit the shear criterion can be used in conjunction with the Mises, Johnson-Cook, Hill, and Drucker-Prager plasticity models, including equation of state. In Abaqus/Standard it can be used with the Mises, Johnson-Cook, Hill, and Drucker-Prager models.

DAMAGE INITIATION, CRITERION=SHEAR, KS=$k_s$, DEPENDENCIES=n

The numerical results obtained using the linear form of damage accumulation described above are not always in good agreement with experimental results. It has been shown that much better agreement can be achieved if a formulation based on a power law rule is used to characterize the nonlinearity in the damage accumulation. This power law formulation is available with the ductile and shear criteria in both Abaqus/Standard and Abaqus/Explicit and with the Johnson Cook criterion in Abaqus/Explicit. For the ductile and Johnson Cook criteria it has the form:

and for the shear criterion it can be expressed as:

where n is the accumulation power.

DAMAGE INITIATION, ACCUMULATION POWER=n

Optionally, you can specify the initial work hardened state of the material by providing the initial equivalent plastic strain values (see Defining initial values of state variables for plastic hardening) and, if residual stresses are also present, the initial stress values (see Defining initial stresses). Abaqus uses this information to initialize the values of the ductile and shear damage initiation criteria, ${\omega }_D$ and ${\omega }_S$, assuming constant values of stress triaxiality and shear shear ratio (linear stress path).

INITIAL CONDITIONS, TYPE=HARDENING
INITIAL CONDITIONS, TYPE=STRESS

Load module: Create Predefined Field: Step: Initial, choose Mechanical for the Category and Hardening and Stress for the Types for Selected Step

The forming limit diagram (FLD) is a useful concept introduced by Keeler and Backofen (1964) to determine the amount of deformation that a material can withstand prior to the onset of necking instability. The maximum strains that a sheet material can sustain prior to the onset of necking are referred to as the forming limit strains. A FLD is a plot of the forming limit strains in the space of principal (in-plane) logarithmic strains. In the discussion that follows major and minor limit strains refer to the maximum and minimum values of the in-plane principal limit strains, respectively. The major limit strain is usually represented on the vertical axis and the minor strain on the horizontal axis, as illustrated in Figure 1. The line connecting the states at which deformation becomes unstable is referred to as the forming limit curve (FLC). The FLC gives a sense of the formability of a sheet of material. Strains computed numerically by Abaqus can be compared to a FLC to determine the feasibility of the forming process under analysis.

Figure 1. Forming limit diagram (FLD).

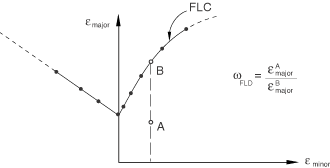
The FLD damage initiation criterion requires the specification of the FLC in tabular form by giving the major principal strain at damage initiation as a tabular function of the minor principal strain and, optionally, temperature and predefined field variables, ${\epsilon }_{\mathrm{major}}^{\mathrm{FLD}}\left({\epsilon }_{\mathrm{minor}},\theta ,f_i\right)$. The damage initiation criterion for the FLD is given by the condition ${\omega }_{\mathrm{FLD}}=1$, where the variable ${\omega }_{\mathrm{FLD}}$ is a function of the current deformation state and is defined as the ratio of the current major principal strain, ${\epsilon }_{\mathrm{major}}$, to the major limit strain on the FLC evaluated at the current values of the minor principal strain, ${\epsilon }_{\mathrm{minor}}$; temperature, $\theta$; and predefined field variables, $f_i$:

For example, for the deformation state given by point A in Figure 1 the damage initiation criterion is evaluated as ${\omega }_{\mathrm{FLD}}={\epsilon }_{\mathrm{major}}^{\mathrm{A}}/{\epsilon }_{\mathrm{major}}^{\mathrm{B}}.$

If the value of the minor strain lies outside the range of the specified tabular values, Abaqus will extrapolate the value of the major limit strain on the FLC by assuming that the slope at the endpoint of the curve remains constant. Extrapolation with respect to temperature and field variables follows the standard conventions: the property is assumed to be constant outside the specified range of temperature and field variables (see Material data definition).

Experimentally, FLDs are measured under conditions of biaxial stretching of a sheet, without bending effects. Under bending loading, however, most materials can achieve limit strains that are much greater than those on the FLC. To avoid the prediction of early failure under bending deformation, Abaqus evaluates the FLD criterion using the strains at the midplane through the thickness of the element. For composite shells with several layers the criterion is evaluated at the midplane of each layer for which a FLD curve has been specified, which ensures that only biaxial stretching effects are taken into account. Therefore, the FLD criterion is not suitable for modeling failure under bending loading; other failure models (such as ductile and shear failure) are more appropriate for such loading. Once the FLD damage initiation criterion is met, the evolution of damage is driven independently at each material point through the thickness of the element based on the local deformation at that point. Thus, although bending effects do not affect the evaluation of the FLD criterion, they may affect the rate of evolution of damage.

DAMAGE INITIATION, CRITERION=FLD

When strain-based FLCs are converted into stress-based FLCs, the resulting stress-based curves have been shown to be minimally affected by changes to the strain path (Stoughton, 2000); that is, different strain-based FLCs, corresponding to different strain paths, are mapped onto a single stress-based FLC. This property makes forming limit stress diagrams (FLSDs) an attractive alternative to FLDs for the prediction of necking instability under arbitrary loading. However, the apparent independence of the stress-based limit curves on the strain path may simply reflect the small sensitivity of the yield stress to changes in plastic deformation. This topic is still under discussion in the research community.

A FLSD is the stress counterpart of the FLD, with the major and minor principal in-plane stresses corresponding to the onset of necking localization plotted on the vertical and horizontal axes, respectively. In Abaqus the FLSD damage initiation criterion requires the specification of the major principal in-plane stress at damage initiation as a tabular function of the minor principal in-plane stress and, optionally, temperature and predefined field variables, ${\sigma }_{\mathrm{major}}^{\mathrm{FLSD}}\left({\sigma }_{\mathrm{minor}},\theta ,f_i\right)$. The damage initiation criterion for the FLSD is met when the condition ${\omega }_{\mathrm{FLSD}}=1$ is satisfied, where the variable ${\omega }_{\mathrm{FLSD}}$ is a function of the current stress state and is defined as the ratio of the current major principal stress, ${\sigma }_{\mathrm{major}}$, to the major stress on the FLSD evaluated at the current values of minor stress, ${\sigma }_{\mathrm{minor}}$; temperature, $\theta$; and predefined field variables, $f_i$:

If the value of the minor stress lies outside the range of specified tabular values, Abaqus will extrapolate the value of the major limit stress assuming that the slope at the endpoints of the curve remains constant. Extrapolation with respect to temperature and field variables follows the standard conventions: the property is assumed to be constant outside the specified range of temperature and field variables (see Material data definition).

For reasons similar to those discussed earlier for the FLD criterion, Abaqus evaluates the FLSD criterion using the stresses averaged through the thickness of the element (or the layer, in the case of composite shells with several layers), ignoring bending effects. Therefore, the FLSD criterion cannot be used to model failure under bending loading; other failure models (such as ductile and shear failure) are more suitable for such loading. Once the FLSD damage initiation criterion is met, the evolution of damage is driven independently at each material point through the thickness of the element based on the local deformation at that point. Thus, although bending effects do not affect the evaluation of the FLSD criterion, they may affect the rate of evolution of damage.

DAMAGE INITIATION, CRITERION=FLSD

Another approach available in Abaqus/Explicit for accurately predicting the forming limits for arbitrary loading paths is based on the localization analysis proposed by Marciniak and Kuczynski (1967). The approach can be used with the Mises and Johnson-Cook plasticity models, including kinematic hardening. In M-K analysis, virtual thickness imperfections are introduced as grooves simulating preexisting defects in an otherwise uniform sheet material. The deformation field is computed inside each groove as a result of the applied loading outside the groove. Necking is considered to occur when the ratio of the deformation in the groove relative to the nominal deformation (outside the groove) is greater than a critical value.

Figure 2 shows schematically the geometry of the groove considered for M-K analysis. In the figure a denotes the nominal region in the shell element outside the imperfection, and b denotes the weak groove region. The initial thickness of the imperfection relative to the nominal thickness is given by the ratio $f_0=t_0^b/t_0^a$, with the subscript 0 denoting quantities in the initial, strain-free state. The groove is oriented at a zero angle with respect to the 1-direction of the local material orientation.

Figure 2. Imperfection model for the M-K analysis.

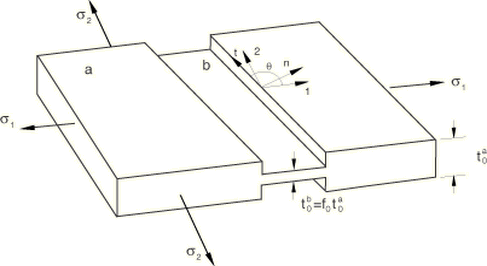
Abaqus/Explicit allows the specification of an anisotropic distribution of thickness imperfections as a function of angle with respect to the local material orientation, $f_0\left(\theta \right)$. Abaqus/Explicit first solves for the stress-strain field in the nominal area ignoring the presence of imperfections; then it considers the effect of each groove alone. The deformation field inside each groove is computed by enforcing the strain compatibility condition

and the force equilibrium equations

The subscripts n and t refer to the directions normal and tangential to the groove. In the above equilibrium equations $F_{nn}$ and $F_{nt}$ are forces per unit width in the t-direction.

The onset of necking instability is assumed to occur when the ratio of the rate of deformation inside a groove relative to the rate of deformation if no groove were present is greater than a critical value. In addition, it may not be possible to find a solution that satisfies equilibrium and compatibility conditions once localization initiates at a particular groove; consequently, failure to find a converged solution is also an indicator of the onset of localized necking. For the evaluation of the damage initiation criterion Abaqus/Explicit uses the following measures of deformation severity:

These deformation severity factors are evaluated on each of the specified groove directions and compared with the critical values. (The evaluation is performed only if the incremental deformation is primarily plastic; the M-K criterion will not predict damage initiation if the deformation increment is elastic.) The most unfavorable groove direction is used for the evaluation of the damage initiation criterion, which is given as

where $f_{eq}^{\mathrm{crit}}$, $f_{nn}^{\mathrm{crit}}$, and $f_{nt}^{\mathrm{crit}}$ are the critical values of the deformation severity indices. Damage initiation occurs when ${\omega }_{\mathrm{MK}}=1$ or when a converged solution to the equilibrium and compatibility equations cannot be found. By default, Abaqus/Explicit assumes $f_{eq}^{\mathrm{crit}}=f_{nn}^{\mathrm{crit}}=f_{nt}^{\mathrm{crit}}=10$; you can specify different values. If one of these parameters is set equal to zero, its corresponding deformation severity factor is not included in the evaluation of the damage initiation criterion. If all of these parameters are set equal to zero, the M-K criterion is based solely on nonconvergence of the equilibrium and compatibility equations.

You must specify the fraction, $f_0$, equal to the initial thickness at the virtual imperfection divided by the nominal thickness (see Figure 2), as well as the number of imperfections to be used for the evaluation of the M-K damage initiation criterion. It is assumed that these directions are equally spaced angularly. By default, Abaqus/Explicit uses four imperfections located at 0°, 45°, 90°, and 135° with respect to the local 1-direction of the material. The initial imperfection size can be defined as a tabular function of angular direction, $f_0\left(\theta \right)$; this allows the modeling of an anisotropic distribution of flaws in the material. Abaqus/Explicit will use this table to evaluate the thickness of each of the imperfections that will be used for the evaluation of the M-K analysis method. In addition, the initial imperfection size can also be a function of initial temperature and field variables; this allows defining a nonuniform spatial distribution of imperfections. Abaqus/Explicit will compute the initial imperfection size based on the values of temperature and field variables at the beginning of the analysis. The initial size of the imperfection remains a constant property during the rest of the analysis.

A general recommendation is to choose the value of $f_0$ such that the forming limit predicted numerically for uniaxial strain loading conditions (${\epsilon }_{\mathrm{minor}}=0$) matches the experimental result.

The virtual grooves are introduced to evaluate the onset of necking instability; they do not influence the results in the underlying element. Once the criterion for necking instability is met, the material properties in the element are degraded according to the specified damage evolution law.

DAMAGE INITIATION, CRITERION=MK, DEPENDENCIES=n

DAMAGE INITIATION, CRITERION=MK, FEQ=$f_{eq}^{\mathrm{crit}}$, FNN=$f_{nn}^{\mathrm{crit}}$,
 FNT=$f_{nt}^{\mathrm{crit}}$

DAMAGE INITIATION, CRITERION=MK,
 NUMBER IMPERFECTIONS=$n_{\mathrm{imp}}$, FREQUENCY= $N_{\mathrm{incr}}$

Müschenborn and Sonne (1975) proposed a method to predict the influence of the deformation path on the forming limits of sheet metals on the basis of the equivalent plastic strain, by assuming that the forming limit curve represents the sum of the highest attainable equivalent plastic strains. Abaqus makes use of a generalization of this idea to establish a criterion of necking instability of sheet metals for arbitrary deformation paths. The approach requires transforming the original forming limit curve (without predeformation effects) from the space of major versus minor strains to the space of equivalent plastic strain, ${\overline{\epsilon }}^{pl}$, versus ratio of principal strain rates, $\alpha ={\dot{\epsilon }}_{\mathrm{minor}}/{\dot{\epsilon }}_{\mathrm{major}}$.

For linear strain paths, assuming plastic incompressibility and neglecting elastic strains:

As illustrated in Figure 3, linear deformation paths in the FLD transform onto vertical paths in the ${\overline{\epsilon }}^{pl}$–$\alpha$ diagram (constant value of $\alpha$).

Figure 3. Transformation of the forming limit curve from traditional FLD representation (a) to MSFLD representation (b). Linear deformation paths transform onto vertical paths.

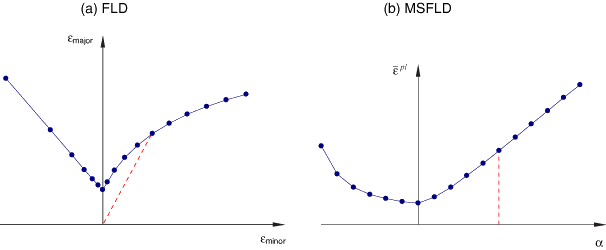
According to the MSFLD criterion, the onset of localized necking occurs when the sequence of deformation states in the ${\overline{\epsilon }}^{pl}$–$\alpha$ diagram intersects the forming limit curve, as discussed below. It is emphasized that for linear deformation paths both FLD and MSFLD representations are identical and give rise to the same predictions. For arbitrary loading, however, the MSFLD representation takes into account the effects of the history of deformation through the use of the accumulated equivalent plastic strain.

For the specification of the MSFLD damage initiation criterion in Abaqus, you can directly provide the equivalent plastic strain at damage initiation as a tabular function of $\alpha$ and, optionally, equivalent plastic strain rate, temperature, and predefined field variables, ${\overline{\epsilon }}_{\mathrm{MSFLD}}^{pl}\left(\alpha ,{\dot{\overline{\epsilon }}}^{pl},\theta ,f_i\right)$. Alternatively, you can specify the curve in the traditional FLD format (in the space of major and minor strains) by providing a tabular function of the form ${\epsilon }_{\mathrm{major}}\left({\epsilon }_{\mathrm{minor}},{\dot{\overline{\epsilon }}}^{pl},\theta ,f_i\right)$. In this case Abaqus will automatically transform the data into the ${\overline{\epsilon }}^{pl}$–$\alpha$ format.

Let ${\omega }_{\mathrm{MSFLD}}$ represent the ratio of the current equivalent plastic strain, ${\overline{\epsilon }}^{pl}$, to the equivalent plastic strain on the limit curve evaluated at the current values of $\alpha$; strain rate, ${\dot{\overline{\epsilon }}}^{pl}$; temperature, $\theta$; and predefined field variables, $f_i$:

The MSFLD criterion for necking instability is met when the condition ${\omega }_{\mathrm{MSFLD}}=1$ is satisfied. Necking instability also occurs if the sequence of deformation states in the ${\overline{\epsilon }}^{pl}$–$\alpha$ diagram intersects the limit curve due to a sudden change in the straining direction. This situation is illustrated in Figure 4. As $\alpha$ changes from ${\alpha }_t$ to ${\alpha }_{t+\mathrm{\Delta }t}$, the line connecting the corresponding points in the ${\overline{\epsilon }}^{pl}$–$\alpha$ diagram intersects with the forming limit curve. When this situation occurs, the MSFLD criterion is reached despite the fact that ${\overline{\epsilon }}_{t+\mathrm{\Delta }t}^{pl}<{\overline{\epsilon }}_{\mathrm{MSFLD}}^{pl}\left({\alpha }_{t+\mathrm{\Delta }t}\right)$. For output purposes Abaqus sets the value of ${\omega }_{\mathrm{MSFLD}}$ equal to one to indicate that the criterion has been met.

Figure 4. Illustration of how a sudden change in the straining direction, from ${\alpha }_t$ to ${\alpha }_{t+\mathrm{\Delta }t}$, can produce a horizontal intersection with the limit curve and lead to onset of necking.

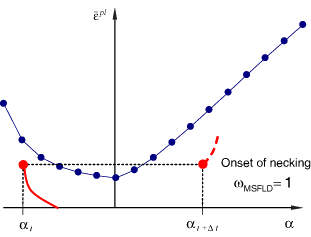
The equivalent plastic strain ${\overline{\epsilon }}^{pl}$ used for the evaluation of the MSFLD criterion in Abaqus is accumulated only over increments that result in an increase of the element area. Strain increments associated with a reduction of the element area cannot cause necking and do not contribute toward the evaluation of the MSFLD criterion.

If the value of $\alpha$ lies outside the range of specified tabular values, Abaqus extrapolates the value of equivalent plastic strain for initiation of necking assuming that the slope at the endpoints of the curve remains constant. Extrapolation with respect to strain rate, temperature, and field variables follows the standard conventions: the property is assumed to be constant outside the specified range of strain rate, temperature, and field variables (see Material data definition).

As discussed in Progressive damage and failure of ductile metals, predictions of necking instability based on the MSFLD criterion agree remarkably well with predictions based on the Marciniak and Kuczynski criterion, at significantly less computational cost than the Marciniak and Kuczynski criterion. There are some situations, however, in which the MSFLD criterion may overpredict the amount of formability left in the material. This occurs in situations when, sometime during the loading history, the material reaches a state that is very close to the point of necking instability and is subsequently strained in a direction along which it can sustain further deformation. In this case the MSFLD criterion may predict that the amount of additional formability in the new direction is greater than that predicted with the Marciniak and Kuczynski criterion. However, this situation is often not a concern in practical forming applications where safety factors in the forming limit diagrams are commonly used to ensure that the material state is sufficiently far away from the point of necking. Refer to Progressive damage and failure of ductile metals for a comparative analysis of these two criteria.

For reasons similar to those discussed earlier for the FLD criterion, Abaqus evaluates the MSFLD criterion using the strains at the midplane through the thickness of the element (or the layer, in the case of composite shells with several layers), ignoring bending effects. Therefore, the MSFLD criterion cannot be used to model failure under bending loading; other failure models (such as ductile and shear failure) are more suitable for such loading. Once the MSFLD damage initiation criterion is met, the evolution of damage is driven independently at each material point through the thickness of the element based on the local deformation at that point. Thus, although bending effects do not affect the evaluation of the MSFLD criterion, they may affect the rate of evolution of damage.

DAMAGE INITIATION, CRITERION=MSFLD, DEFINITION=MSFLD

DAMAGE INITIATION, CRITERION=MSFLD, DEFINITION=FLD

DAMAGE INITIATION, CRITERION=MSFLD, PEINC=${\left(\mathrm{\Delta }{\overline{\epsilon }}^{pl}\right)}^{*}$, 
OMEGA=$\omega$

Property module: material editor: MechanicalDamage for Ductile MetalsMSFLD Damage: Omega: $\omega$

INITIAL CONDITIONS, TYPE=HARDENING
INITIAL CONDITIONS, TYPE=PLASTIC STRAIN

Load module: Create Predefined Field: Step: Initial, choose Mechanical for the Category and Hardening for the Types for Selected Step