Overview of Reliability Analysis Methods

Methods for structural reliability analysis have been developed to incorporate uncertainties associated with geometrical and material properties, loading and boundary conditions, and operational environment into structural analysis and design. These uncertainties are incorporated through the definition of random variables, their associated probabilistic distribution functions, and statistical properties. Given one or more identified random variables, the focus in structural reliability analysis is to assess the probability of failure—the probability of violating a constraint—of a structural component or system, resulting from performance (output) variation caused by the variation of uncertain, random (input) variables. The structural reliability is then defined as the probability of satisfying a constraint and is equal to 1– the probability of failure.

The concepts of reliability and probability of failure are shown in the following figure:



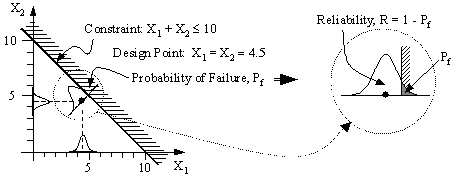
For the example shown above the current design point, given as X1=X2=4.5, is within the specified constraint of X1+X2≤10: therefore, this design appears to be feasible. If both X1 and X2 are known to vary around their nominal values (roughly between values of 3 and 6 in the figure above), the performance measure Y=X1+X2 will also vary. In this case a portion of the expected distribution of Y lies outside the constraint. The area of this distribution that is outside the constraint defines the probability of failure associated with this design point and the defined constraint. The area of the distribution that lies within the constraint (in the feasible region) is defined as the reliability level of this design.

Many methods for determining the probability of failure or reliability (estimating the areas inside and outside the constraints) have been developed in recent years. These methods are used to evaluate reliability of the current design point.